Discover the Fascinating World of Foundations of Discrete Harmonic Analysis: Applied and Numerical Harmonic

Discrete harmonic analysis is a branch of mathematics that deals with the study of signals and functions in a discrete setting. It provides a systematic framework for analyzing and understanding the underlying structure of signals and data. In this article, we will explore the foundations of discrete harmonic analysis, its practical applications, and the numerical techniques used for harmonic analysis.

The Basics of Discrete Harmonic Analysis

Discrete harmonic analysis builds upon the concepts of classical harmonic analysis, but focuses on the analysis of signals and functions defined on discrete sets, such as digital images, time series data, and audio signals. The key idea is to expand a given signal or function into a sum of discrete harmonic components, similar to how a Fourier series represents a periodic function as a sum of sine and cosine functions.

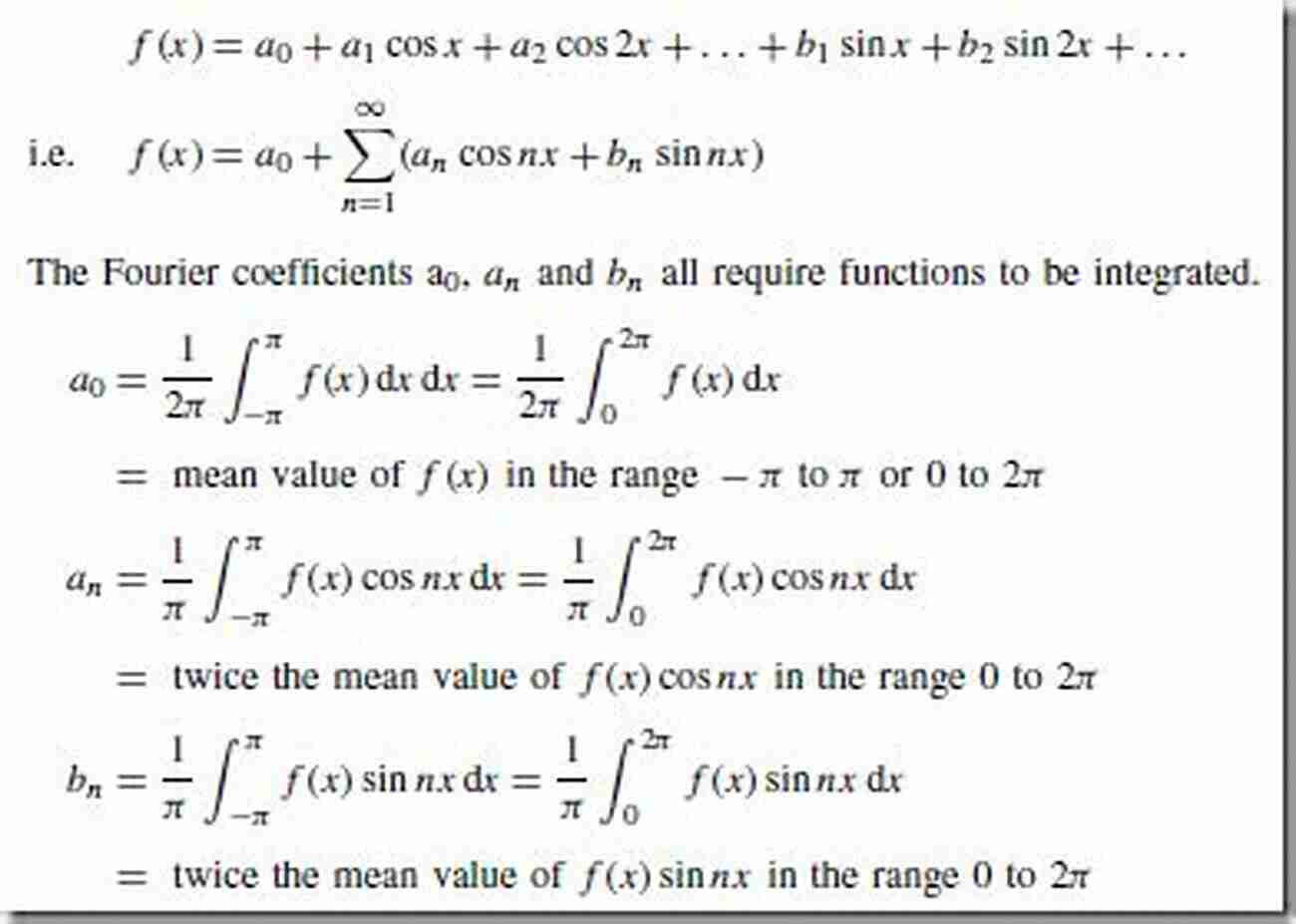
The foundations of discrete harmonic analysis lie in the notion of discrete Fourier transform (DFT),which allows us to decompose a discrete signal or function into its frequency components. The DFT is a powerful tool for analyzing various properties of signals, including their energy distribution, spectral content, and periodicity.

Foundations of Discrete Harmonic Analysis (Applied and Numerical Harmonic Analysis)

by Paul B. Yale(1st ed. 2020 Edition, Kindle Edition)

4.3 out of 5

Language	:	English
File size	:	4713 KB
Print length	:	262 pages
Screen Reader	:	Supported

FREE

Practical Applications of Discrete Harmonic Analysis

The principles of discrete harmonic analysis find numerous applications in various fields, including signal processing, image analysis, data compression, audio and video coding, and communication systems. Let's delve into some of these applications:

1. Signal Processing:

Discrete harmonic analysis plays a fundamental role in signal processing tasks such as noise filtering, audio equalization, speech recognition, and image enhancement. By decomposing a signal into its frequency components using techniques like the DFT, we can isolate and manipulate specific frequencies to achieve the desired processing goals.

2. Image and Video Analysis:

In image and video analysis, discrete harmonic analysis techniques are employed for tasks like image compression, image recognition, and video object tracking. Transform-based methods, such as the discrete cosine transform (DCT) and discrete wavelet transform (DWT),exploit the frequency content of images and videos to achieve efficient compression and accurate feature extraction.

3. Data Compression:

By exploiting the redundancy present in signals and data, discrete harmonic analysis techniques enable efficient data compression. For instance, JPEG image compression uses the DCT to remove redundant high-frequency components, resulting in a compact representation without significant loss of visual quality.

Numerical Techniques for Harmonic Analysis

When dealing with real-world signals and data, we often face practical challenges like noise corruption, limited sampling, and computational efficiency. To tackle these challenges, various numerical techniques have been developed in the field of discrete harmonic analysis.

1. Fast Fourier Transform (FFT):

The FFT is an efficient algorithm for computing the DFT and its inverse. It exploits the symmetry and periodicity properties of the DFT to reduce the computational complexity from O(n^2) to O(n log n),where n is the signal length. The FFT has revolutionized many fields, enabling real-time signal processing, fast image compression, and rapid solving of partial differential equations.

2. Wavelet Transform:

The wavelet transform is a powerful technique that allows for both frequency and time localization of signal components. It decomposes a signal into a set of wavelets, localized oscillatory functions with variable frequency and duration. The wavelet transform is extensively used in image denoising, transient signal analysis, and pattern recognition tasks.

3. Sparse Approximation:

Sparse approximation methods aim to represent a signal or data using only a few significant coefficients. These methods leverage the sparsity property of signals, i.e., the fact that most real-world signals can be well approximated using only a small number of basis functions or features. Techniques like compressive sensing and sparse representation have gained popularity in various applications, including image reconstruction, sensor array processing, and source localization.

Foundations of discrete harmonic analysis provide a powerful framework for understanding and manipulating signals and data in a discrete setting. By decomposing signals into their frequency components, we can gain insights into their structure, extract useful information, and achieve efficient data compression. Moreover, numerical techniques like the fast Fourier transform and wavelet transform enable fast and accurate computation of harmonic analysis. Whether it's image processing, signal analysis, or data compression, discrete harmonic analysis plays a crucial role in enhancing our understanding and manipulation of digital signals in various domains.

Foundations of Discrete Harmonic Analysis (Applied and Numerical Harmonic Analysis)

by Paul B. Yale(1st ed. 2020 Edition, Kindle Edition)

4.3 out of 5

Language	:	English
File size	:	4713 KB
Print length	:	262 pages
Screen Reader	:	Supported

FREE

This book provides an to discrete harmonic analysis (DHA) with a view towards applications to digital signal processing. In a nutshell, DHA is used to determine the time-frequency structure of a digitized signal, providing a representation of the signal as a sum of spectral components that can then be analyzed.

The main methods of DHA are discrete Fourier transform and other discrete orthogonal transforms such as the Walsh and Haar transforms. Fast algorithms are used to process signals in real time, while additional options are provided by spline harmonic analysis. These topics are carefully covered in the book. With only modest prerequisites, some of which are recalled at the beginning, a profound mathematical theory is built almost from scratch. The 150 exercises included form an integral part of the text.

Based decades of teaching experience, this book provides a basis for lecture courses starting at the upper undergraduate level, and will also prove a valuable resource for mathematicians and engineers interested in digital signal processing.